Defining Place Value: The 6 aspects
Mar 03, 2022
The six aspects of place value
My experience as a teacher, leader and researcher in Years 3-6 classrooms has led me to believe that many of our students can be described as ‘apparent experts’ in place value. These students appear to understand place value, yet often their knowledge falters with deeper questioning. For example, if I asked how many hundreds, tens and ones are in 375 and to represent the number using Base 10 blocks, they can do so confidently. However, if asked to represent the number in another way, for example 37 tens and 5 ones, they look at me blankly.
As teachers, we all appreciate how important place value is- it is like the frame of a house- it holds everything else up. A student who lacks a robust understanding of place value is hindered in all aspects of mathematics, particularly in Years 3-6. From my research it has become clear that the first step to improve the teaching and learning of place value is to concisely define place value. Once we have a shared framework for teachers to follow, our teaching in place value across the school can be structured and focused.
If we look at the current Australian Curriculum: Mathematics (2022) descriptors, there are 11 verbs related to place value teaching and learning (model, read, write, count, recognize, represent, order, group, partition, rearrange and regroup). Most teachers I have worked with have a good understanding of what it means to teach modelling, reading, writing, counting, recognizing, representing and ordering numbers. However, things become a little sketchy when teachers are asked to describe grouping, partitioning, rearranging and regrouping. Comments like: “oh I call that partitioning” or “I thought that was rearranging” are common.

I think this lack of consistency is a major issue for our teaching of place value. If we don’t have agreement on what we are teaching or what language we are using to describe it, how can we teach in a consistent manner across our school? Imagine a student who is learning about regrouping in Year 3, and then suddenly in Year 4 the same action is referred to as rearranging. Surely this is confusing!
In order to address this, one of the first tasks I tackled in my PhD research was to define place value. I read LOTS and LOTS of research around everything related to place value. I ended up with 6 categories, or as I call them aspects to describe the content we need to cover when teaching place value.
The diagram below shows the 6 aspects.
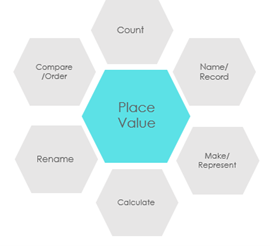
Rogers (2014)
I will now take each of the six aspects and briefly define them.
Name/Record
Definition: Read and write a number in words and symbols (e.g., ‘seventy-five’ and 75). Name the place value columns (the hundreds column is next to the tens column). Round numbers to the nearest place value part (567 is closest to 6 hundreds). Determine the value of a digit (the digit 7 in 75 has the value 70)
Count:
Definition: Counting forwards and backwards in place value parts (e.g., 45, 55, 65 is counting using the unit ten). Bridging forwards and backwards over place value segments such as decuples and centuples (e.g., 995 and one more ten requires bridging forwards over hundreds to thousands). Applying language such before, after, between, more, less.
Make/Represent:
Definition: Make, represent or identify the value of a number using a range of materials or manipulatives. These may be proportional (e.g., base-ten blocks), non-proportional (e.g. coloured counters) and be presented as canonical (e.g., 3 tens and 9 ones is 39) or non-canonical (e.g., 2 tens and 19 ones is 39) representations.
Compare/Order:
Definition: Compare numbers to determine which is larger or smaller. Compare numbers in a multiplicative manner, for example ten times larger than 54 is 540. Place numbers in descending or ascending order and locate numbers on empty, partially marked or complete number lines.
Rename:
Definition: Rename numbers in multiple ways in terms of place value parts without the use of manipulatives (e.g., 1 260 is equivalent to 126 tens or 12 hundreds and 6 tens or 1 thousand and 260 ones).
Calculate
Definition: Apply knowledge and understanding of the place value system when completing calculations using the four operations (e.g., 45 multiplied by ten is 45 tens, 45 plus 100 is 145, 120 divided by ten is 12)
These 6 aspects guide my planning, teaching and assessment of place value. The most important change for me was rolling grouping, partitioning, rearranging and regrouping together into the ‘rename’ aspect. I think this change alone will greatly help improve the consistency of our approach to place value. The beauty of the six aspects is that they are applicable for decimal and whole number place value, so they literally can form the perfect base from which to build a whole school approach to place value.
If you would like to learn more about using the six aspects in your assessment and teaching of place value, you might be interested in my place value mini course. You can find out more here.
Want to learn more from Dr Ange Rogers? Click here to find out about her 'Quality Place Value Assessment in Years 3-6 Mini Course'



